Python Exercises: Iterated Cube Root

Last update on April 17 2025 13:00:37 (UTC/GMT +8 hours)

Cube Root Reduction Steps

Write a Python program that takes a positive integer and calculates the cube root of the number until the number is less than three. Count the number of steps to complete the task.

Sample Data:

(3) -> 1
(39) -> 2
(10000) -> 2

Sample Solution-1:

Python Code:

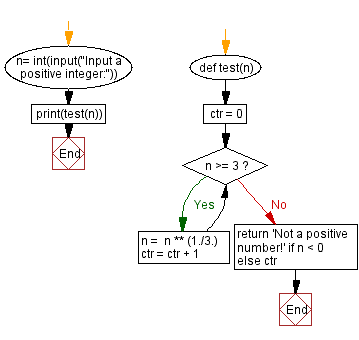
# Function to count the number of times a positive integer can be cubed until it is less than 3.
def test(n):
    # Counter variable initialized to 0.
    ctr = 0
    
    # Loop continues until n is greater than or equal to 3.
    while n >= 3:
        # Cube root of n is calculated using the expression n ** (1./3.).
        n = n ** (1./3.)
        
        # Increment the counter by 1 for each iteration.
        ctr = ctr + 1
    
    # Return 'Not a positive number!' if the final value of n is negative, otherwise, return the counter value.
    return 'Not a positive number!' if n < 0 else ctr

# Take user input for a positive integer.
n = int(input("Input a positive integer:"))

# Call the test function with the user-input value of n and print the result.
print(test(n))

Sample Output:

Input a positive integer: 3
1
Input a positive integer: 39
2
Input a positive integer: 10000
2
Input a positive integer: -4
Not a positive number!

Explanation:

Here is a breakdown of the above Python code:

Function definition:

def test(n):: Defines a function named "test()" that takes a positive integer 'n' as input.

Counter Initialization:

ctr = 0: Initializes a counter variable to 0.

While Loop:

while n >= 3:: A while loop that continues as long as the value of n is greater than or equal to 3.

Cube root calculation:

n = n (1./3.): Calculates the cube root of n using the expression n (1./3.).

Counter Increment:

ctr = ctr + 1: Increments the counter by 1 for each iteration of the loop.

Return statement:

return 'Not a positive number!' if n < 0 else ctr: Returns 'Not a positive number!' if the final value of 'n' is negative; otherwise, returns the counter value.

User input:

n = int(input("Input a positive integer:")): Takes user input for a positive integer.

Function call and print:

print(test(n)): Calls the "test()" function with the user-input value of 'n' and prints the result.

Flowchart:

Sample Solution-2:

Python Code:

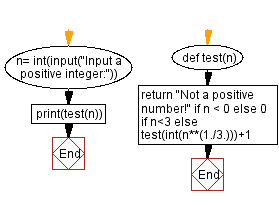
# Recursive function to count the number of times a positive integer can be cubed until it is less than 3.
def test(n):
    # Return "Not a positive number!" if n is negative.
    if n < 0:
        return "Not a positive number!"
    
    # Return 0 if n is less than 3.
    elif n < 3:
        return 0
    
    # Recursive call to test function with the cube root of n, and add 1 to the result.
    else:
        return test(int(n ** (1./3.))) + 1

# Take user input for a positive integer.
n = int(input("Input a positive integer:"))

# Call the test function with the user-input value of n and print the result.
print(test(n))

Sample Output:

Input a positive integer: 14
1
Input a positive integer: -5
Not a positive number!

Explanation:

Here is a breakdown of the above Python code:

Function definition:

def test(n):: Defines a recursive function named "test()" that takes a positive integer n as input.

Conditional statements:

if n < 0:: Checks if 'n' is negative and returns "Not a positive number!" in that case.
elif n < 3:: Checks if 'n' is less than 3 and returns 0 in that case.

Recursive call:

return test(int(n ** (1./3.))) + 1: Recursively calls the "test()" function with the cube root of n and adds 1 to the result.

User input:

n = int(input("Input a positive integer:")): Takes user input for a positive integer.

Function Call and Print:

print(test(n)): Calls the "test()" function with the user-input value of 'n' and prints the result.

Flowchart:

For more Practice: Solve these Related Problems:

Write a Python program to repeatedly take the cube root of a number until the result is less than three, counting the steps.
Write a Python program to implement a loop that computes the cube root of an integer until the result falls below three, and count the iterations.
Write a Python program to reduce a number by taking its cube root repeatedly until it becomes less than three, outputting the step count.
Write a Python program to calculate how many cube root operations are needed for a number to drop below three.

Go to:

Previous: N x N square consisting only of the integer N
Next: Python String Exercise Home.

Python Code Editor:

Have another way to solve this solution? Contribute your code (and comments) through Disqus.